Research Article
Theory and Experiments. (+) Add Reading Glasses to Prevent Myopia

Peter R Greene1* and Brown OS2
1B.G.K.T. Consulting Ltd. Bioengineering Huntington, New York
2Optics C & O Research Waynesboro, Penn
*Address for Correspondence: Peter R Greene, B.G.K.T. Consulting Ltd. Bioengineering Huntington, New York 11743, Tel: +1 631 935 56 66; E-mail: [email protected]
Dates: Submitted: 26 October 2016; Approved: 16 February 2017; Published: 20 February 2017
How to cite this article: Greene PR, Brown E EOS. Theory and Experiments. (+) Add Reading Glasses to Prevent Myopia. Int J Clin Exp Ophthalmol. 2017; 1: 009-022. DOI: 10.29328/journal.ijceo.1001002
Copyright Licence: © 2017 Greene PR, et al. This is an open access article distributed under the Creative Commons Attribution License, which permits unrestricted use, distribution, and reproduction in any medium, provided the original work is properly cited.
Keywords: Progressive myopia; Reading glasses; Bifocals; Progressive add lenses (pals); Refraction; Myopia prevention; Diopters
Abstract
Basic control theory equations are developed showing conventional exponential system response of refraction vs. time R (t) with a characteristic system time constant, in response to a step change of near work environmental conditions. Details from preliminary experimental design using reading glasses at the U.S. Naval Academy at Annapolis are discussed. The conclusion is that (+) add lenses, used as reading glasses during study, can prevent the development of myopia for college students in pilot training.
Introduction
Literature Review: Cheng et al. [1,2] and Gwiazda et al. [3,4], present a comprehensive listing of modern studies to date in terms of using (+) Add reading glasses to prevent or slow the progression of myopia. Brown & Berger [5], Brown & Young [6], Schaeffel & Howland [7], Medina & Fariza [8], and Greene, Brown, Medina & Graupner [9] use first order control theory to predict myopia development as a function of time. Thorn, Gwiazda & Held [10], present a mathematical model of myopia development using the Gompertz function. Hung & Ciuffreda [11], develop IRDT, incremental retinal defocus, to explain myopia during the growth phase. Medina et al. [12-14] and Greene & Medina [15-18], use control theory to explain myopia development, solved with digital and analog computer techniques to evaluate first-order equations. Viikari [19] and Goldschmidt [20], present comprehensive reviews of juvenile myopia, and various techniques used to control this myopia progression.
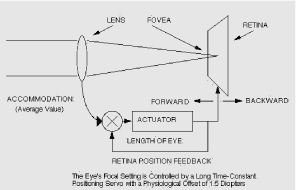
Theories are many and varied, in terms of the causes of myopia [19,20]. Figure 1a and 1b below show the near work demand problem, typical of college students, and the proposed optical solution to the problem, namely, custom reading glasses [21]. In this report, various mathematical control theories are reviewed, derived from conventional electrical engineering concepts, as shown in figure 1c below. When a strong negative change is made in your visual environment, normal eyes will change their focal state as shown figure 1d below.
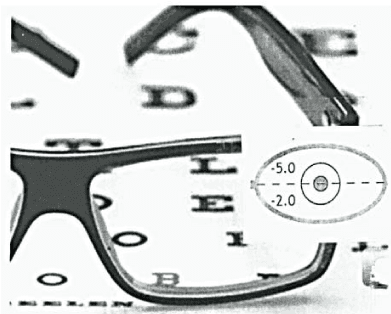
Figure 1a: Norman Rockwell’s “The Law Student”, from the Saturday Evening Post, is seen reading at an effective distance of -3.0 to -4.0 diopters.
Figure 1b: Reading glasses for a -5.00 D. college myope. (+) Add technology is used by both bifocals and progressive addition lenses, “PAL’s”. PAL’s are “no-line” bifocals. Basically, these (+) Add reading glasses are distance compensators, with a +3.00 D Add for reading.
The eye will also show a similar response to a strong positive change in its environment. There is -- and this is important -- a limit to the amount by which you can change your visual environment in a positive direction.
Figure 1e below shows the correlation between the eye’s focal status [diopters] and the Snellen eye chart acuity as measured, from 20/20 to 20/120:
Materials and Methods
The Naval Academy requires unaided visual acuity of 20/20 in each eye as a basic entrance requirement. Prospective pilots are required to have normal vision on graduation. A substantial number of midshipmen, entering with 20/20 vision, become myopic during their four years at the Academy. A reasonable assessment of the experimental evidence suggests that the eye sets its long-term focus by a servo control process. This engineering analysis of the eye’s control action predicts that a significant percentage of midshipmen could avoid the myopia problem is they wear a convex lens while reading.
Focal Stability Considerations: An engineering approach to the problem of the eye’s long-term focus leads to the considerations of two major forms of focal control: genetic and servo [22]. The initial heredity postulate was described by Stieger, reviewed by Brown [23]. This theory suggests that all optical components of focus are controlled by genetic information. The assumption of this theory is that the eye ignores the accommodation signal while growing. This approach leads to severe design difficulties when the eye’s focal status is considered from a cybernetic point of view.
Measurements made by Dr. Young have shown that major optical components vary by factors of twenty percent over the growing period. These focal components of the eye change in an apparently random manner [24]. We should expect to find long-term focal variations of twenty percent (eight diopters) drifting from one extreme to the other-assuming that the postulate of genetic (open-loop) control is correct. In addition, this randomness should exist between the left and right eye, creating occasional differentials of sixteen diopters. Measurements of the eye’s actual focal status do not confirm such variations. A theoretical measurement study indicates that the eye has a tracking probable error of approximately 1/10 diopters [25].
A Focal Servo: A systems analysis approach to the design of a mechanism that is comparable to the eye shows that it is possible to account for the eye’s focal accuracy, providing the system is feedback controlled. The concept that heredity is a factor in the eye’s servo design is an appropriate postulate. Servo control, however, is the fundamental design factor in a mechanism that is comparable to the eye in its response to focal perturbations.
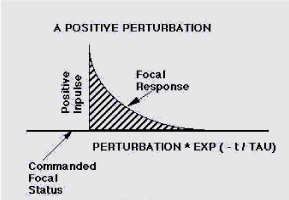
Focal Noise: There are many sources of focal perturbations. These sources include temperature changes, pressure changes, and extrinsic muscle pulls on the sclera [26]. A perturbation is a small plus/minus change in the eye’s focal status (Figures 2). A purely genetic theory predicts that the eye will not initiate corrective action in the presence of focal noise.
System Design: Since the goal of the eye’s design is to achieve stability in the presence of noise, the eye does not need a fast acting second-order servo to maintain focal accuracy. It is unlikely that the system will have a complex transfer function. The most probable design is a servo with a very slow velocity constant. A system of this design will have the following transfer function.
1/ [(TAU s + 1) s], where Time Const. TAU= 100 Days
Knowing the form of the transfer function allows us to experimentally determine the system’s time constant. This has been done with adolescent pigtail macaque monkeys [27]. The eye’s time domain response (long-term focus) is given by the equation:
Focus= (Heredity offset) + Accommodation + Delta Accommodation * [1 - EXP (-t / TAU)] [7]
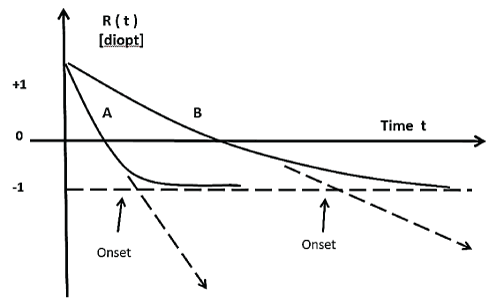
A step change in the value of accommodation will produce a time constant response in the eye’s focal status (Figure 2a).
The Offset: The existence of a fixed offset is a requirement of the focal servo design. The system is designed for normal variation in the average value of accommodation. The eye’s offset and time constant prevent the eye from becoming myopic due to a confined visual environment of short duration. It is reasonable to believe that the offset is a function of the individual’s heredity. A review of the experimental evidence shows and estimated value of +1.5 diopters for the offset.
A Piecewise Equation: In a previous paper, we developed a piecewise (replacement) equation for the eye’s focal status [28].
Focus= (Offset + Accommodation - Focus) / TAU + Focus - Perturbation
This equation allows the continuous calculation and prediction of the eye’s focal status, providing the eye’s average value of accommodation is known on a daily basis. The focal status predictions of this equation have been experimentally verified by experimental work with monkeys [27,28].
A Design Limitation. The existence of a long-term focal servo insures focal accuracy. The eye’s visual environment is normally between -0.2 to -1.0 diopters. We will define this range of values as an “open” visual environment. A focal servo that obeys this equation will have a design limitation. The eye should not be placed in an environment that is “nearer” that about -1.0 diopters. There are individual differences in the heredity offset which make it difficult to calculate precisely how much confined visual environment an individual can tolerate before he becomes nearsighted.
If we wish to sustain normal vision, it is essential that we be aware of this limitation, and the consequence of exceeding this visual environment for long periods of time. Unfortunately, the school environment imposes requirements that force us to exceed this limitation. The result of an increasingly confined environment on the eye’s focal servo is a gradual change in focal status from hyperopia into myopia. By recognizing this limitation, we can initiate a preventive effort to neutralize the “near” environment before myopia actually becomes a problem.
Changing the Environment with a Lens: Since the focal status of a servo controlled eye is locked to the average value of accommodation, shifts in the accommodation, shifts in the accommodation signal will result in corresponding shifts in the eye’s focal status. A convex lens may be used to substantially alter the average value of accommodation-thus allowing us to maintain the eye in an “open” visual environment.
A positive lens will simulate the effect of a distant object on the eye’s accommodation system. Prolonged use of this lens for all close work will prevent the development of myopia in the servo model. This neutralization effect of the positive lens has been experimentally demonstrated during a four year bifocal study conducted by Oakley and Young [29] (Figure 3a,b).
While this analysis is simplified, it suggests the nature of what can be done to keep the eye in a normal focal condition with a convex lens. Even if a large amount of hyperopic reserve has been lost, our analysis shows that it can be slowly restored by the assiduous use of a convex lens for all close work.
The preceding considerations are the basis for the second opinion on the care and control of myopia. A thumbnail survey of the actual practice of eye care shows the following breakdown as to the preferred procedure for dealing with the problem of incipient nearsightedness.
1. Approximately eighty percent will use a negative lens to achieve immediate restoration of 20/20 vision.
2. Approximately twenty percent will use the positive (bifocal) lens to lessen the impact of a confined visual environment.
3. Less than one percent will use an assortment of techniques, including prisms to neutralize convergence, and atropine to paralyze the accommodation system.
It is clear that these two opinions will not be resolved in the immediate future by experiment, theory, or practice. The normal approach used in the fact of disputed evidence and unresolved opinions is to explain both approaches to the individual-allowing him to make the final decision regarding the use of myopia avoidance techniques [1-4,14-17,29].
Since the loss of hyperopic reserve is a clear signal of the impending onset of nearsightedness, the time to make the decision about myopia avoidance is before the eye actually fails the eye chart.
The avoidance technique requires both and persistence. To support the effort, the individual should be given information that will provide an intellectual understanding of the rationale for the use of the preventive lens.
Since the effort must be maintained for a long period of time to show the full effect of the avoidance technique, it will take an individual with a personal interest in retaining normal vision to habitually use the plus for all close work. For this reason, we believe that prospective pilots who are almost into myopia will be particularly interested in the program.
Sustained myopia avoidance by use of a convex lens is a very difficult goal to achieve. There are many subtle problems in such an effort. It will take a sophisticated level of understanding to:
1. Recognize nearsightedness as a design limitation of the normal eye.
2. Recognize the necessity of maintaining the eye in an artificially open environment with a positive lens- for as long as the eye is kept in a confined environment.
In the past it has been easier to do nothing in the early stage of myopia, and to wear a corrective lens to restore 20/20 vision at a later date. Since the plus lens has no instant effect, other than an apparent lessening of eye strain, there is a tendency to discount the long-term effect of a convex lens.
Partial efforts with a positive lens that does not completely neutralize the near environment will be marginally successful. A servo analysis suggests that conscientious wearing of a positive lens that fully neutralizes the near environment will result in a slow restoration of a normal amount of hyperopic reserve.
Since success of the preventive effort depends on a coherent understanding of the eye’s normal control action, it would be advisable to build a fully functioning camera that sets its long-term focus as described in this paper-thus providing a valuable instructional aid for those involved in the program.
Our eyes function so well that we tend to ignore the existence of a design limitation. We expect perfection at all times . . . so much so that we ignore the fact that our eyes obey principles that a designer would use to build a similar device. The servo nature of the normal eye would be recognized if it were a fast acting mechanical device. Our evaluation is that the eye functions as a very slow acting servo system. The fact that this biological devices is constructed of collagen and other biomaterials tends to obscure this characteristic of the eye’s design.
Results
Because the eye is sophisticated in its design and operational characteristics, the engineering requirement for focal accuracy suggests that the eye sets its long-term focus by a servo control process. The mechanism that establishes the eye’ focal status consists of two separate systems. The first system is a blur driven accommodation mechanism that regulates the focal power of the eye’s lens for maximum image sharpness at the surface of the retina. This control system has a time constant of 0.25 seconds [25].
The second system, which is responsible for controlling the eye’s long-term focus, has a time constant of 100 days [27,28,30]. In a normal visual environment the eye’s focal status will be from 0 to +2 diopters. The focal servo system functions to overcome the inevitable perturbations that occur within the eye’s optical system [5,6]. This system has a tracking probable error of 1/10 diopters [25].
Since the focus of a servo controlled eye is coupled to the average value of accommodation, shifts in the accommodation signal will result in corresponding shifts in the eye’s focal status. This predictable consequence of the design has been experimentally verified [5,6].
The Eye’s Focal Transfer Function. Servo systems are coupled systems that are defined by their transfer function. We have tentatively established the fact that the normal eye’s behavior obeys the following transfer function [5,6]: 1 / (TAU s + 1).
The normal eye’s response to a step function is found by:
System’s Response= [(Step Function) / (s)] * [1 / (TAU s + 1)]
The eye’s time domain response to this step function is:
Focus= Offset + Accommodation + Delta * [1 - EXP (- t / TAU)]
This equation has been verified by applying a step function to the visual environment of monkeys [19] and by then measuring the resulting focal status change at two week intervals for a year.
A servo system will exhibit a response to noise (perturbations) that exists within the control loop. These random focal changes will cause the system to initiate corrective shifts in the eye’s focal status. The eye’s time domain response to an impulse perturbation is:
Focus= Offset + Accommodation - Perturbation * EXP (- t / TAU)
These two equations predict the eye’s response to two idealized inputs. They do not yield explicit predictions if the eye’s visual environment is changing by significant amounts.
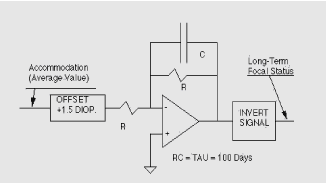
A Piece-Wise Time Domain Equation: We may obtain a piece-wise (iterative) equation for the 1 / (TAU s + 1) transfer function by reviewing a block diagram of the eye’s focal control system (Figure 3c).
The input for this system is the command signal: (Equation 1 below)
Eq. 1: Commanded Focal Status = Offset + Accommodation (Daily Average)
The error signal is: (Equation 2 below)
Eq. 2: Error Signal = Commanded Focal Status - The eye’s Actual Focal Status
Since the actuator has a very slow velocity constant (K), the amount of error correction achieved in one day is given by: (Equation 3 below)
Eq. 3: Focal Servo Change = Error Signal / TAU
Using this equation, we may calculate the effect of each day’s value of accommodation on the eye’s focal status: (Equation 4 below)
Eq. 4: Updated Focal Status = Yesterday’s Focal Status + Focal State Change
Including the effects of random perturbations, the equation becomes, combining equations 1, 2, 3, and 4 (Equation 5 below)
Eq. 5: Updated Focus = [(Offset + Accommodation-Focus) / TAU] + Focus - Perturbation
For clarity of analysis in this chapter we will set the perturbation level of the eye to zero diopters. In a normal eye, focal noise and measurement errors produces a measured tracking error of 1/10 diopters [25]. The equation allows the prediction of the eye’s focal status on a continuing basis. Due to the long time-constant of the system, each day’s average value of accommodation will have a very small effect on the focal status of the normal eye.
The piece-wise equation provides an alternative method for solving the 1 / (TAU s + 1) transfer function. The input for the equation is the signal that is obtained from the accommodation system which is identical to your visual environment.
Equation Verification: The piece-wise equation, in this form, predicts the same results that were obtained for a step function change in accommodation and an impulse perturbation on the eye’s focal status [5,6].
The Hereditary Offset: The hereditary offset is a latent constant of vision (Figure 3d).
If a group of individuals have a constant visual environment of -0.5 diopters, their eyes will show a range of focal status values of from zero to two diopters. The mean hereditary offset for the group is +1.5 diopters.
If the average visual environment is changed from -0.5 diopters to -1.5 diopters, the focal status of all individuals will change towards a negative focal state (myopia), as predicted by the equation./p>
The Average Value of Accommodation: The controlling variable for this equation is the eye’s value of accommodation. The focal settings of the lens is determined by information decoded at the surface of the retina. The visual environment may be calculated by the use of the equation:
VISUAL ENVIRONMENT = - 1 / (OBJECT DISTANCE)
Eq. (6)(In Diopters) (In Meters)
A visual object moved inwards from infinity to one meter constitutes an environment change of - 1.0 diopters. Under this circumstance, the accommodation system will servo the lens by + 1.0 diopters to again achieve sharp focus at the surface of the retina.
Average Visual Environment: The average value of accommodation can be determined if an individual’s environment is known on a daily basis. If the individual spends 8 hours outdoors (0 diopters) and 8 hours reading (-3.0 diopters) his average visual environment will be -1.5 diopters.
A Normal Physiological System: This equation was developed to explain the high level of focal accuracy that is measured in the normal human and primate eye.
We know of few qualitative, and no quantitative theories that can explain focal accuracy of 1/10 diopters for the normal eye. In the absence of any other focal control equation that can provide a logical explanation for such precision, we tentatively propose that this equation and model accurately represents the normal eye’s behavior.
Many theories have been developed to explain myopia (as a defect of the eye). Most of these theories suggest a failure mode in genetics, convergence, or mechanical structure that ultimately results in nearsightedness. We feel it is premature to discuss these theories until we have a clear understanding of the fundamental behavioral characteristic of the normal eye. We will, therefore, examine the design limitation of a normal feedback controlled eye.
Normal-Eye Myopia. We may define two major environments for this system.
1. A normal visual environment of from -0.2 diopters to -0.5 diopters.
2. A confined visual environment of from -1.5 diopters to -2.0 diopters.
If an individual with a hereditary offset of +1.0 diopters uses his eyes in an open environment for a long time, his focal status will be approximately defined by the equation:
Focus= Offset + Accommodation - Perturbation * EXP (- t / TAU)
Using typical values:
Focus= + 1.0 + (-.5) - 0 * EXP (- t / TAU)
Focus= + 0.5 Diopters
This individual will have 20/20 vision with a positive focal state (hyperopic) of + 0.5 diopters [5,6].
This generalized analysis has been confirmed by measurements made by Dr. Young on adult “hunting” Eskimos [24]. If this individual maintains his eyes in a confined visual environment, his eyes will show a slow ramp into myopia. After two hundred days his focal status will be:
Focus= +1.0 + (-1.5) - (0) * EXP (- t / TAU)
Focus= - 0.5 diopters
The same individual, who had 20/20 vision in a normal visual environment, now has 20/40 vision with a focal status of -0.5 diopters. A prolonged confined environment violates a design constraint of the human and primate eye. We will define nearsightedness that develops in this way as servo-myopia. This is entirely normal behavior for a normal eye.
Design Limitation: This analysis suggests that the normal eye will avoid serving into nearsightedness if the eye is maintained in an “open” visual environment. Obviously this goal conflicts with the requirement that we spend prolonged hours at close work for the ten to twenty years that we spend in school.
The effect of a confined environment can be neutralized by the use of a convex (plus) lens. A convex lens is a converging lens. Parallel rays of light (from infinity) are brought to a point by this lens. The converse relationship is true. Diverging rays of light from a nearby object will be made parallel by the use of a convex lens. If reading is done at the focal point of the lens, the value of accommodation will be 0 diopters, rather than -2.0 diopters without the lens (Figure 3b).
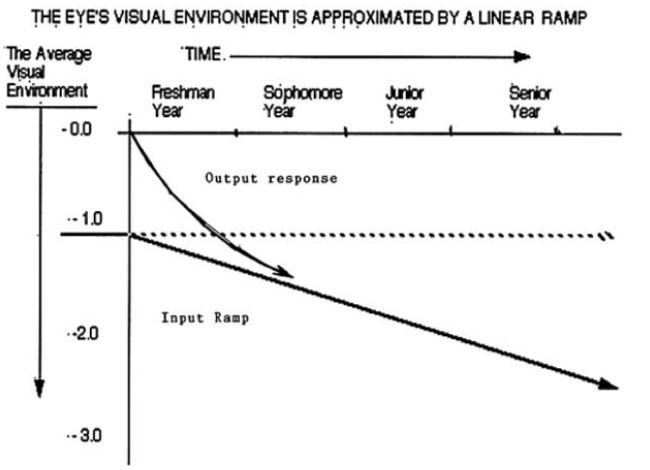
Visual Environment of College Students: As we enter higher academic institutions, our visual environment gradually shifts to a more negative value, figure 1a. We can characterize this increased “near” environment by the following ramp function: A = m t + b
A= m t + b, where:
A= Accommodation (daily average value) from the start of the freshman year.
m= -0.001 diopters / day = -0.365 D/yr
t= time in days
b= -1.0 diopters
The Laplace transform of a unit ramp is: 1 / (s^2)
Applying this ramp to the eye’s transfer function produces: System’s Response = [m / s ^2] * [1 / (TAU s + 1)]
The eye’s time domain response to a ramp function is [30]:
Focus= Offset Accommodation (Initial Value) (Ramp) + Accommodation * TAU [(t / TAU) - 1 + EXP (- t / TAU)]
Discussion
Focal Status Produced by a Plus Lens: After two hundred days, this equation predicts that the eye will show the same linear slope as the accommodation ramp. We can logically expect that the eyes of college students will show a gradual movement towards, and eventually, into myopia when a linear ramp is applied to their accommodation system.
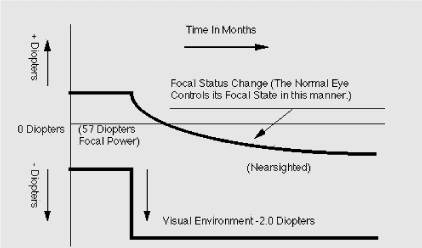
How close does this scenario match the actual situation? In a study of the cadets at West Point, Dr. Gmelin determined that freshmen with 20/20 vision and 0 diopters focal state would, after four years, develop 20/80 vision with -1.3 diopters of myopia [31]. A similar study was conducted at the United States Naval Academy by Dr. Hayden, reviewed by Greene et al. [15,16,32,33] (2015, 2016). This study showed an approximately linear change in focal status towards myopia in the eyes of almost all the normal eyed midshipmen [28,30,31].
Figure 1a shows Norman Rockwell’s classic painting “The Law Student”, from the Saturday Evening Post, where the student is seen reading at an effective distance of -3.0 to -4.0 diopters.
Myopia Prevention. We have plotted the historical development of myopia as a function of time (Figure 4). The dynamic theory explicitly states that the eyes of the midshipmen move into nearsightedness due to their increasingly confined visual environment. Their focal status change is in the right direction and proper magnitude to suggest quantitative verification for this dynamic model of the normal eye’s long-term behavior.
If their myopia is a result of normal servoing action, a major means of changing this situation would be to use a convex lens for all close work. Use of this lens would substantially alter their visual environment from an estimated value of -1.5 diopters, to a more reasonable figure of -0.4 diopters. Their resulting focal status was calculated by the use of the equation developed [5,6,30].
Conclusion
Work done during the past twenty years has demonstrated that the accommodation system is a superb example of a physiological control system. It is a complex, sophisticated, and accurate system. We can logically expect that the normal eye will show equal competence in the design of its long-term control system.
The process of building a mathematical model of a physiological system necessarily implies the idealization of that system. Models are not tested in a vacuum. The development of a servo-heredity model suggests an alternative model which we will call, for the purpose of identification and discussion, a “negative-lens-heredity” theory of the eye’s long-term focus.
Heredity is a fundamental factor in both of these theories. There is a disagreement on how this factor establishes the eye’s long-term focus. These theories yield contradictory predictions that can be tested. The negative-lens theory predicts that:
1. The eye’s focal status will not change due to a plus or minus change in the eye’s visual environment.
2. A plus or minus lens has no effect on the eye’s long-term focus. Nearsightedness can not be produced in a normal eye by the prolonged wearing of a minus lens.
3. The change in focal status of students towards myopia is not related to their increasingly confined visual environment. The development of myopia is due to the genetic make-up of the individual.
A number of these stated predictions have been tested. We feel that the current experimental evidence more strongly supports the concept that the eye is servo controlled versus the concept that the eye is not servo controlled. Actual myopia prevention is a very difficult task to accomplish. This demonstrates that effective myopia prevention is a reasonable expectation, provided the convex lens is assiduously used for all close work. The belief that this approach will work is reflected in current eye care practices [1-4,19-21,30-35]. About twenty percent of the profession will use the plus lens (bifocal) to deal with the problem of incipient myopia.
Appendix I Continuous Correction Myopia Ramp
This Appendix derives the observed “linear myopia ramp”, frequently reported in the experimental and clinical literature [36], Medina (2015).
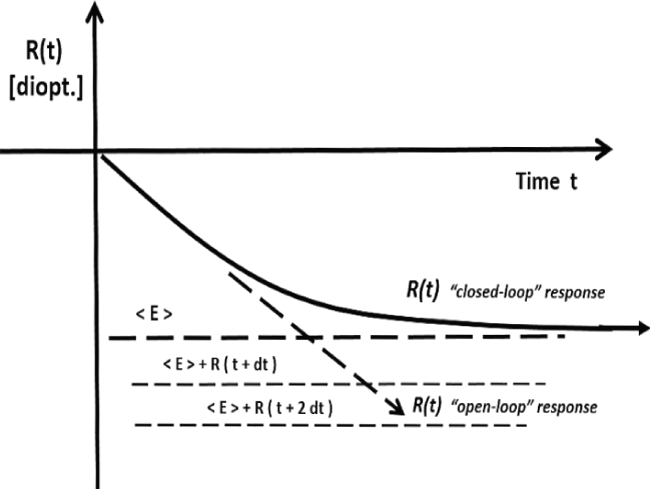
The proof that “continuous” or “continual” correction of myopia produces a linear drift of refraction, equivalent to “open-loop” drift, is proven as follows Ordinarily, the 1-st order closed-loop system response to a negative step
(A-1) R (t) = Ro + (Ro -
(A-2a) dR(t)/dt = k [ R(t) -
(A-2b) dR(t)/dt = ( 1 / τ ) [ R(t) - (
Note that the current refraction R (t) cancels out in (A-2b), and thus there is no feedback, an open-loop system.
These equations are integrated, with Eq. (A-2a) resulting as Eq. (A-1) above and Eq. (A-2b) resulting in the observed linear ramp function
(A-3) R (t) = Ro - k
Where Ro is the initial refraction at time to,
Perhaps the most interesting feature of these results is that the system time constant τ (typically τ=0.25 to 1.0 years) appears in both the closed-loop exponential and open-loop linear equations A-1 and A-3 above. In other words, the progressive myopia ramp-rate, Eq. A-3, is given by R’ = -
Conclusion
These results predict that those individuals with rapid emmetropization time constant τ, will also demonstrate rapid myopia rates
Acknowledgement
Partial support for this work was provided by NIH NEI Grant EY 005013 at the Depts. of Biomedical Engineering and Ophthalmology at Johns Hopkins University. The authors would like to thank Jacob Raphaelson, Frank Young, David Guyton, Antonio Medina, Karel Montor and Sterling Colgate for many helpful discussions.
References
- Cheng D, Woo GC, Drobe B, Schmid KL. Effect of bifocal and prismatic bifocal spectacles on myopia progression in children: three-year results of a randomized clinical trial. JAMA Ophthalmol. 2014; 132: 258-264. Ref.: https://goo.gl/E789xz
- Cheng D, Woo GC, Schmid KL. Bifocal lens control of myopic progression in children. Clin Exp Optom. 2011; 94: 24-32. Ref.: https://goo.gl/mqvTn1
- Gwiazda J, Hyman L, Hussein M, Everett D, Norton TT. et al. A randomized clinical trial of progressive addition lenses versus single vision lenses on the progression of myopia in children. Invest Ophthalmol Vis Sci. 2003; 44: 1492-1500. Ref.: https://goo.gl/U6U0cH
- Scheiman M, Zhang Q, Gwiazda J, Hyman L, Harb E. Visual activity and its association with myopia stabilisation. Ophthalmic Physiol Opt. 2014; 34: 353-361. Ref.: https://goo.gl/3IDylC
- Brown O, Berger R. A Nearsightedness Computer, Proceedings of the Annual New England Bioengineering Conference pp. 343-346, 1979.
- Brown OS, Young FA. The response of a servo controlled eye to a confined visual environment. Biomed Sci Instrum. 1981; 17: 41-44. Ref.: https://goo.gl/RU5185
- Schaeffel F, Howland HC. Mathematical model of emmetropization in the chicken. J Opt Soc Am A. 1988; 5: 2080-2086. Ref.: https://goo.gl/2mhfKv
- Medina A, Fariza E. Emmetropization as a first-order feedback system.Vision Res. 1993; 33: 21-26. Ref.: https://goo.gl/Yl2yKN
- Greene PR, Brown OS, Medina AP, Graupner HB. Emmetropia approach dynamics with diurnal dual-phase cycling. Vision Res. 1996; 36: 2249-2251. Ref.: https://goo.gl/zCDEJR
- Thorn F, Gwiazda J, Held R. Myopia progression is specified by a double exponential growth function. Optom Vis Sci. 2005; 82: 286-297. Ref.: https://goo.gl/SAZfAJ
- Hung GK, Ciuffreda KJ. Incremental retinal-defocus theory of myopia development--schematic analysis and computer simulation. Comput Biol Med. 2007; 37: 930-946. Ref.: https://goo.gl/KY2CeU
- Medina A. The progression of corrected myopia. Graefes Arch Clin Exp Ophthalmol. 2015; 253: 1273-1277. Ref.: https://goo.gl/afeSBy
- Medina A, Greene PR. Progressive Myopia and Lid Suture Myopia are Explained by the Same Feedback Process: a Mathematical Model of Myopia. J Nat Sci. 2015; 1. Ref.: https://goo.gl/SbOsGA
- Medina A. Detecting the effect of under-correcting myopia. Graefes Arch Clin Exp Ophthalmol. 2016; 254: 409-410. Ref.: https://goo.gl/rZK2Iz
- Greene PR, Grill ZW, Medina A. Mathematical Models of College Myopia. Optik (Stuttg). 2016; 127: 896-899. Ref.: https://goo.gl/oEluRM
- Greene, PR, Medina A. Analogue Computer Model of Progressive Myopia–Refraction Stability Response to Reading Glasses. J Comput Sci Syst Biol. 2016: 104. Ref.: https://goo.gl/ome9Xp
- Greene, PR, Medina A. The Progression of Nearwork Myopia. Optom Open Access. 2016: 2. Ref.: https://goo.gl/irX5Bv
- Greene PR, Grill ZW, Medina A. Exploring Reading Glasses to Stabilize College Myopia, J. Sci. Med. Ophthalmology. 2015; 3: 1034-1039. Ref.: https://goo.gl/wvVcVE
- Viikari, K. Learn to understand & prevent myopia. BoD-Books on Demand. 2011. Ref.: https://goo.gl/IxBLFb
- Goldschmidt E. The mystery of myopia. Acta Ophthalmol Scand. 2003; 81: 431-436.Ref.: https://goo.gl/J9b9TT
- Nan, L, Seger K, Crandall, M, & Jiang, B. C. et al. Effect of customized near addition lenses on transient myopia induced by near-work. Clinical Optometry. 2010; 2: 43-49. Ref.: https://goo.gl/ZYBeQe
- Brown O, Young F, Physiological Modeling: The Long-Term Growth of the Eye, Proceedings of the 8th New England Bioengineering Conference.1980; 133-136.
- Brown OS. How to Avoid Nearsightedness, C & O Research, Penn., Library of Congress #89-91632, 2004; ISBN #0-917882-30-X. Ref.: https://goo.gl/7nW2Gf
- Young FA, Leary GA, Baldwin WR, West DC, Box RA, et al. The transmission of refractive errors within eskimo families. Am J Optom Arch Am Acad Optom. 1969; 46: 676-685.Ref.: https://goo.gl/FTAHTn
- Hooker RJ, Brown PR, Greene JS, Moore, Colgate SA. Analog computer simulation of accommodation. In Engineering in Medicine and Biology Society, 1996. Bridging Disciplines for Biomedicine. Proceedings of the 18th Annual International Conference of the IEEE, vol. 5, pp. 1777-1779. IEEE.
- Greene, PR, Sergienko, NM, Wang SK. Review: Measurement Techniques for Intraocular Pressure. Optom Open Access. 2016; 118: 2. Ref.: https://goo.gl/H9RVBh
- Young FA. The effect of restricted visual space on the primate eye. Am J Ophthalmol. 1961; 52: 799-806. Ref.: https://goo.gl/BT8leb
- Brown O, Young F, Berger R. Measuring the Eye’s Focal Accuracy-A Heuristic Approach. IEEE Transactions on Biomedical Engineering 1981 Jan 1, Vol. 28, No. 8, p. 581-581. Ref.: https://goo.gl/133UgQ
- Oakley KH, Young FA. Bifocal control of myopia. Am J Optom Physiol Opt. 1975; 52: 758-764. Ref.: https://goo.gl/lbxVPi
- Brown OS, Young FA, Shotwell AJ. A predictive mathematical model for the eye's focal status. Biomed Sci Instrum. 1982; 18: 111-116. Ref.: https://goo.gl/jKWWdF
- Gmelin RT. Myopia at West Point: past and present. Mil Med. 1976; 141: 542-543. Ref.: https://goo.gl/GDB2A7
- Greene PR, Vigneau ES, Greene J. Exponential prevalence and incidence equations for myopia. Clin Exp Optom. 2015; 98: 210-213. Ref.: https://goo.gl/4vHttu
- Greene PR, Medina A. Refraction data survey: 2nd generation correlation of myopia. Int Ophthalmol. 2016; 36: 609-614. Ref.: https://goo.gl/poZbla
- Fledelius HC. Myopia profile in Copenhagen medical students 1996-98. Refractive stability over a century is suggested. Acta Ophthalmol Scand. 2000; 78: 501-505. Ref.: https://goo.gl/mHZWXF
- Lin Z, Vasudevan B, Mao GY, Ciuffreda KJ, Jhanji V, et al. The influence of near work on myopic refractive change in urban students in Beijing: a three-year follow-up report. Graefes Arch Clin Exp Ophthalmol. 2016; 254: 2247-2255. Ref.: https://goo.gl/OQAyKO
- Goss DA. Linearity of refractive change with age in childhood myopia progression. Am J Optom Physiol Opt. 1987; 64):775-780. Ref.: https://goo.gl/PbyaV5